I have discovered that Yin-Yang and Golden Ratio are different representations of one and the same metaphysical principle. The metaphysical principle of our interest is Unity Within Duality which states the complementary character of conflicting tendencies. This is of course similar to the Hegelian trinity in his interpretation of history: Thesis-Antithesis-Synthesis.
After giving a short account of Yin-Yang and Golden Ratio I proceed to prove their equivalence. Since after a year of research I have found no one else who has discovered this equivalence I stick a copyright notice at the end of the article just for the publication purposes in case the finding turns out to be of significance.
Yin-Yang and Golden Ratio are the two representations of the principle that maximally opposing tendencies, or aspects, complement one another to form a unity: The former represents it geometrically and the latter represents it numerically.
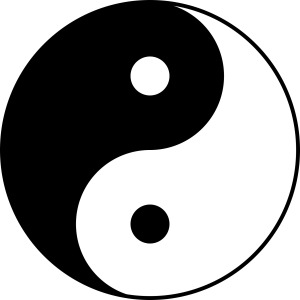
Yin-Yang in Chinese philosophy is the geometrical expression of the following concept: The apparently opposing and contradictory tendencies complement one another; being interconnected they add up to form a unity. In the Yin-Yang symbol a whole circle is divided into two maximally opposing aspects. The two aspects enjoy maximal opposition both in color and orientation: The color of one cut is the negation of the color of the other; and the orientation of one is the inverse of the orientation of the other.
Notice that the two opposing sides have the same shape; they are the opposites of one another only in the attributes color and orientation. In other words, one side is the negation and inversion of the other side. But this cannot be done with just any shape; not every shape can be inverted and then added to itself to form a perfect circle which represents unity.
Golden Ratio is a number in mathematics; it has the numerical value of 1.61803398875…. When the ratio of two quantities is this number we say that they are in golden ratio. This number has huge significance in mathematics because it is the corner stone of spiral geometry which is observed everywhere in nature. It is also the limit of the Fibonacci Sequence. In fact when the ratio of two lengths is the golden ratio they have an inherent tendency to make up harmonious and beautiful structures.
It is time to see how Yin-Yang and Golden Ratio are equivalent to one another, both being the representations of one principle.
We saw that Yin-Yang represents the idea that maximally opposing aspects add up to form a unity; each aspect is both the negation and the inversion of the other aspect. Numerically speaking, one being the inverse of the negative of the other. Let us try to represent this idea numerically, using number instead of shape: We need a number that when maximally opposed to itself, namely first negated and then inverted, can be added to itself to form a unity which is represented by number 1.
We don’t yet know if such number exists, but it will soon be proved that it actually does. First remember that by negation of a number of course we mean its negative, -2 being the negative of 2. By inversion we mean the inverse of the number, 1/2 being the inverse of 2; thus, the inverse of the negative of 2 is -1/2; but notice that when we add 2 and -1/2 we get 3/2 and not 1. We are after a number that gives us 1.
Let us call our desired number x and try to write down the concept behind Yin-Yang in the language of numbers and see if such number exists: We have x as our number; negate it to get -x; invert the negation to get -1/x. To express Yin-Yang is to add up these two numbers and have them equal 1. Thus, the algebraic equivalent of Yin-Yang would be the equation [x+(-1/x)]=1 which can be simplified as the following:
X – (1/X) = 1
This already looks like Yin-Yang :). For those with mathematics background this equation should blow their minds; it is none but the infamous equation that is the very definition of the Golden Ratio: Golden Ratio is the exact solution, and the only solution, of this quadratic equation. You will soon see that the second solution is just the negative of the inverse of the Golden Ratio, its maximally opposing complement which we call the Golden Complement. Let’s call this equation, the algebraic form of Yin-Yang, the Golden Equation since its solutions are the Golden Ratio and the Golden Complement; thus, this equation has two solutions in real numbers:
X – 1/X = 1 The Golden Equation (Equivalent of Yin-Yang in Algebra)
First Solution: X1 = 1.61803398875… The Golden Ratio
Second Solution: X2 = -0.61803398875… The Golden Complement
X1 + X2 = 1 The Golden Equation in terms of its two complementary solutions
The first solution is the Golden Ratio and the second solution is interestingly the inverse of the negative of the first solution, namely the Golden Complement. See that the two solutions corresponding to the two complementary aspects of Yin-Yang add up to unity, hence completing the Yin-Yang equivalent in algebra. Notice that the Golden Complement has the exact same decimals as the Golden Ratio itself which is the reason why the decimals cancel out in the Golden Equation, hence leading to unity.
We saw that there actually exists a unique number whose inverse of its negation can be added to it to become unity, the number 1, hence expressing the Yin-Yang principle in the realm of numbers, and this unique number is none but the Golden Ratio, the solution of the Golden Equation. Thus one side of Yin-Yang corresponds to the Golden Ratio and the other side corresponds to its maximally opposing counterpart, the negative of its inverse which is the Golden Complement, and the two adding up to unity by definition. Only the Golden Ratio has this property, and one can see as we showed above that the principle behind Yin-Yang, leading to the Golden Equation in algebra, is the very definition of the Golden Ratio.
Digression:
{Notice that the two solutions of the Golden Equation, which correspond to the two maximally opposing aspects of Yin-Yang, have these two interesting properties:
X1 + X2 = 1 X1 × X2 = -1
If these two solutions, the Golden Ratio and the Golden Complement, are considered to be the diagonal elements, or eigenvalues, of a 2×2 matrix called the Golden Matrix G, then G is an orthogonal matrix with determinant -1 and trace 1, hence:
Det(G) + Tr(G) = 0
Which is only an interesting observation besides our main point.}
End of Digression
Therefore, the principle that the maximally opposing tendencies complement one another and form a unity is represented geometrically as Yin-Yang and algebraically as the Golden Equation X-1/X=1 whose two solutions, corresponding to the two aspects in Yin-Yang, are the Golden Ratio and the Golden Complement. We saw that of all numbers one and only one, the Golden Ratio, has this property. More precisely, the Golden Equation X-1/X=1 is the algebraic equivalent of Yin-Yang from which the Golden Ratio is extracted.
Yin-Yang is the Golden Equation in geometry. Golden Equation is Yin-Yang in algebra.
This equivalence between Yin-Yang and Golden Equation which I discovered in the fall of 2013 has blown my mind to this day. Who could guess that the Golden Ratio is Yin-Yang in numbers while Yin-Yang is the Golden Ratio in geometry! Fascinating.
*[Note for the mathematician: In constructing the Golden Equation, the algebraic equivalent of Yin-Yang, I assumed a bijection from plane shapes to real numbers, hence defining the equation over R, the field of real numbers. Naturally a bijection from the inverse and the negative of a shape would fall onto the inverse and negative of numbers. Thus, in constructing the bijection from Yin-Yang to real numbers the only proper way of translating maximal opposition, namely both negation and inversion, would be to use both the additive inverse and the multiplicative inverse of a number, the only possible ways of defining opposites among real numbers, hence the field properties of the Commutative Division Ring R. In other words, the only way to translate the relation between the two opposing aspects of Yin-Yang is to relate a real number to the multiplicative inverse of its additive inverse, hence mapping the two different operations present in Yin-Yang, namely the spatial inversion and color-negation. It is seen that the only number whose multiplicative inverse of its additive inverse can be added to itself to become unity is the Golden Ratio.]
Copyright © Tomaj Javidtash and NOEMAYA, 12/30/2014
Unauthorized use and/or duplication of this material without express and written permission from this blog’s author and/or owner is strictly prohibited. Excerpts and links may be used, provided that full and clear credit is given to Tomaj Javidtash and NOEMAYA with appropriate and specific direction to the original content.

Reblogged this on Conversations I Wish I Had and commented:
I hope the author will allow me to reblog an article I thought was fascinating. I hope others will be able to appreciate it as much as I did. 🙂
LikeLiked by 2 people
I just saw your message Lyla; it is absolutely fine and I appreciate it very much. Thanks for sharing it and happy new year 🙂
LikeLike
You lost me in the math there, but I think I can hear the music anyway. 😉
LikeLiked by 1 person
🙂
LikeLike
Although I’m a little bit over my head with this, I know it to be true in my heart because mathematics, well science in general, is the concrete expression of spiritual reality. God consciousness is provable as is God. At least I believe that to be true. I don’t think I will be involved in that proving but I know certain things from meditation. Another wonderful article, Toomajj. I’m going to share it with my husband who has a passion for mathematics!!
LikeLiked by 1 person
Thanks 🙂 It is my pleasure.
LikeLiked by 1 person
dear Javid.thanks.god bless u. kindly take up subjects related to human suffering and its solution without much money and pain that is to take every one towards nondual state.you may like the link http://www.spanda.org/origin.html which may help look deep into opposing forces but in unity.
LikeLike
Can you update to include the law of entropy? It’s the same as well.
LikeLiked by 1 person
Thank you for your comment Kayn. I’ve considered that and will update soon.
LikeLike
dear Tomaj , we who are relieved of much pain caused by ignorance wish you a very healthy 2015.
HAPPY NEW YEAR>
LikeLiked by 1 person
Dear Partha, Thanks for your comment. I too wish you a happy and wonderful new year filled with grace and blessings and above all knowledge of Self.
Happy New Year
LikeLike
Excellent way to start a new year!
To see the Universe in a grain of sand.
To see pattern where no thought existed.
–Historian.00
LikeLiked by 1 person
Fascinating argument, Tomaj. All I know is that a structure built according to the golden ratio feels more aesthetically pleasing than other structures, similarly to the “feel” of the yin-yang symbol. I’ve studied the metaphysics of numbers a bit and know that in ancient times, people knew a lot more about numbers and their significance than we do now (e.g. the number one is geometrically represented by a point; it represents wholeness and undifferentiatedness). Keep up the good work, you’ve got a great mind at your disposition.
LikeLiked by 1 person
Thanks for your comment and very kind words. I’m fascinated with numbers as they were studies in ancient times.
LikeLiked by 1 person
I read Pratibha Eastwood’s book “The Nine Windows to Wholeness” which was utterly amazing – never saw numbers in quite the same way again afterwards 🙂
LikeLiked by 1 person
That’s great. I’m gonna give it a read. Thanks for mentioning it.
LikeLiked by 1 person
You are welcome. Thanks for reminding me of the special nature of numbers…
LikeLiked by 1 person
Is there an equivalence of Yin Yang and Golden Section in three dimensions? Have you heard of tribonacci? Does it have any signifigance?
LikeLike
Ummmm, I don’t get it.
I didn’t see any reference to your >>proof<< of equivalence between
any geometrical aspect of taiji (yin yang) and phi
While one might consider black/white a negation, what might be the inversion ?
What geometric aspects are related by a quadratic equation ?
Browne does some cool stuff with taiji variations in multiple dimensions…
http://eprints.qut.edu.au/15012/
The basic radial/linear relations in yinyang are:
r is radius of outer circle
r/2 is radius of inner curves
r/8 is radius of eyes
pi is length of perimeter of each half
pi/2 is the area of each half – unless one wants to treat the pi/64 eyes separately somehow
Where is phi in all that pi and 2^n ?
http://www.goldennumber.net/what-is-phi/
LikeLiked by 1 person
Thanks for leaving your comment here Kurt. I am not aware if someone has referenced my article and and what they have claimed of it. I haven’t really made a mathematical proof of anything but pointed to a conceptual relationship or similarity between the Yin Yang and Golden Ratio. Conceptual and not arithmetical in the sense of the numerical values involved in a circle don’t matter and have no relation to golden ratio number.
It is more of a Platonic work in which I see the idea of unity and duality within it is projected in in geometry as Yin Yang and projected in arithmetic as golden ratio. Here is the gist of it:
Taking the metaphysical idea of unity, its manifestation in the world of numbers is the number 1. Its manfiestation in the world of shapes and geometry is a circle. Not saying that circle and number 1 are the same thing but that both are manifestations of one and the same metaphysical concept of unity.
Now, in case of a circle if want to break this unity into a duality of identical but inverted shapes, the only thing we can produce is Yin Yang since it is breaking the unity of the circle into two identical but inverted shapes. I am not considering the holes here as they are irrelevant.
Now if we go into arithmetic and pick the field of Real numbers: If we want to break number 1 as the sum/addition of two identical but inverted numbers (here inversion translates into possible operations on Real numners which are negation and inversion) then the only number will be golden ration, i.e. if we take the golden ration number and add it to its inverted negative, then the result would be number 1, i.e. unity.
So conceptually, what Yin Yang amounts to is what the algebraic presentation of golden ration (x+(-1/x))=1. That is it. There is no real mathematical utility in this and no grand metaphysical claim. It was just a point out of a conceptual relationship between the two. If you wanna break arithmetical unity into two parts that are reflections/inversions of one another, golden ration would be the only number that does it.
I hope my explanation helped 🙂
LikeLike
Toomajj,
I appreciate your logic of the relationship between the yin/yang and the golden mean. I too am deep into such thinking. I have recently made a discovery dealing with the fibonacci sequence (that is closely related to phi or the golden mean) that amazingly produces the yin/yang as well. These 24 numbers repeat into infinity as the fib sequence does:
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24
1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9
Please email me and I will share you more.
LikeLike
The solutions I’m getting for your equation are X = 1, -1. Where are you getting the golden complement and ratio from? I set the equation to zero and it looks nothing like the equation which yields phi on wikipedia. What gives?
LikeLike
The equation is x-(1/x)=1. Why would you change the 1 to zero! It’s only changing it to zero that you get 1 and -1, but that’s using the incorrect equation. If you keep the equation as it is, and multiply both sides by an x, you’d get a quadratic equation x^2-x-1=0 which has two roots, the golden ratio and it’s golden complement.
LikeLike
Very nice explanation. I was thinking about spiritual stuff and golden ratios and I came across your blog. Anyway, I highly recommend cassiopaea.org which deals mainly with the historical and conceptual aspect of spirituality. Not sure if it will blow your mind but it sure did to mine 🙂
LikeLiked by 1 person
I would have never thought to discover someone else discovering this notion and mathematically expand on it. However I must admit I happened upon the when I was just a kid. And it came to me in a completely and entirely different way. Through geometric shapes. Please email wouldn’t love to expand on it if you’d like:)
LikeLike
I was just thinking to myself, how would I write the equation of the tao in terms of ratio of portion of r as a function of theta. I decided to get some internet help, and this article was the perfect place to start! Thank you!
LikeLiked by 1 person
I’m glad you found this helpful! Your comment made me interested in thinking about the polar form of it which I haven’t yet considered.
LikeLike